Scientists have found a new property of scattering amplitudes based on their investigation of sound waves via solid matter. Details of their findings have been reported in the journal Physical Review Letters.
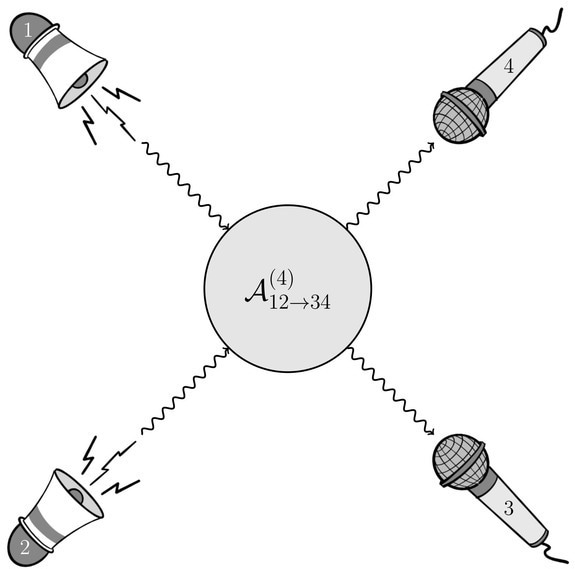Two sound waves (1 and 2) are produced, scattering into two other sound waves (3 and 4), which are then detected. The probability for this process is described by a 4-point scattering amplitude. Image Credit: Angelo Esposito.
Whether it is sound or light, physicists look into the probability of particle interactions concerning scattering amplitudes or probability curves. It is a common myth that, when the energy or momentum of one of the scattered particles reaches zero, scattering amplitudes should scale at all times with integer powers of momentum (i.e., p1, p2, p3, etc.). What the study team discovered, however, was that the amplitude can correspond to a fractional power (i.e., p1/2, p1/3, p1/4, etc.).
What is the significance? While quantum field theories, like the Standard Model, enable scientists to make estimations concerning particle interactions with great accuracy, there is still a chance to enhance the existing basics of fundamental physics.
When a new behavior is revealed—such as fractional-power scaling—researchers obtain the opportunity to review or revisit current theories.
This study, carried out by Angelo Esposito (Institute for Advanced Study), Riccardo Penco (Carnegie Mellon University), and Tomáš Brauner (University of Stavanger), particularly looks into the interactions of sound waves in solids. To imagine this idea, picture a wooden block having speakers positioned on both ends.
Once the speakers are connected to a power supply, two sound waves—phonons—encounter each other and disperse, akin to collisions in a particle accelerator. One speaker is tuned to a specific limit in such a way that the momentum of the phonon is zero, and the subsequent amplitude can be proportional to a fractional power.
This scaling property, the researchers explain, is not likely restricted to phonons in solids, and understanding it may help the exploration of scattering amplitudes in many diverse contexts, spanning from particle physics to cosmology.
The detailed properties of scattering amplitudes have recently been studied with much vigor. The goal of this broad program is to classify possible patterns of behavior of scattering amplitudes, to both make some of our computations more efficient, and more ambitiously, to build new foundations of quantum field theory.
Angelo Esposito, Study Corresponding Author, Institute for Advanced Study
For a long time, Feynman diagrams have been an essential instrument for particle physicists, but they come with a few restrictions. For example, extremely accurate calculations can necessitate tens-of-thousands of Feynman diagrams to be loaded into a computer, to define particle interactions.
By obtaining a proper understanding of scattering amplitudes, scientists can more effortlessly identify particle behavior rather than depending on the top-down method of Feynman diagrams, thus improving the efficacy of calculations.
The present work reveals a twist in the story, showing that condensed matter physics displays much richer phenomenology of scattering amplitudes than what was previously seen in fundamental, relativistic physics. The discovery of fractional-power scaling invites further work on scattering amplitudes of collective oscillations of matter, placing solids in the focus.
Angelo Esposito, Study Corresponding Author, Institute for Advanced Study
Journal Reference:
Brauner, T., et al. (2022) Fractional Soft Limits of Scattering Amplitudes. Physical Review Letters. doi.org/10.1103/PhysRevLett.128.231601.