Aug 11 2020
A new study conducted by the University of Warwick has put a new twist on a mathematical analogy that involves a jumping grasshopper and the perfect shape of its lawn. The study could help interpret the spin states of quantum-entangled particles.
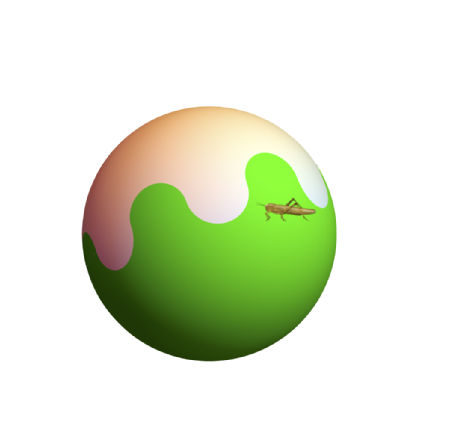
Image Credit: University of Warwick
Physicists Olga Goulko (formerly at the University of Massachusetts at Amherst), Adrian Kent, and Damián Pitalúa-García (Cambridge) devised the grasshopper problem.
The team asked for the perfect lawn shape that would increase the possibility that a grasshopper, beginning from an arbitrary position on the lawn and leaping a fixed distance in a haphazard direction, smoothly lands back on the lawn.
One may intuitively anticipate the answer to be a circular lawn, at least for small leaps. However, Goulko and Kent demonstrated that numerous shapes from a cogwheel pattern to a few detached patches of the lawn performed better for different sizes of jumps.
Beyond surprises regarding grasshoppers and the shapes of lawns, the study offered a valuable understanding of bell-type inequalities concerning probabilities of the spin states of a pair of isolated quantum-entangled particles.
The Bell inequality was first demonstrated by physicist John Stewart Bell in 1964 and subsequently generalized in several ways. It showed that no combination of traditional concepts with Albert Einstein’s unique relativity can describe the hypotheses (and subsequent real experimental observations) of quantum theory.
The following step involves testing the grasshopper issue on a sphere. The Bloch sphere can be described as a geometrical representation of the state space of one quantum bit. On the Bloch sphere, a great circle delineates linear polarization measurements, which are easy to implement and often employed in various cryptographic tests, including the Bell test.
Due to the antipodal symmetry of the Bloch sphere, a lawn conceals 50% of the overall surface area, and the natural theory would be that the perfect lawn is hemispherical in shape. A research team from the department of computer science at the University of Warwick, in association with Goulko and Kent, analyzed this issue and determined that it also needs non-intuitive lawn patterns.
The major outcome of the study is that the hemisphere is never optimal, excluding the unique case when the grasshopper precisely requires a uniform number of jumps to go around the equator. The study demonstrated that there are previously unfamiliar kinds of bell inequalities.
Geometry on the sphere is fascinating. The sine rule, for instance, looks nicer for the sphere than the plane, but this didn’t make our job easy.
Dmitry Chistikov, Study Co-Author and Professor, Department of Computer Science, University of Warwick
Dmitry Chistikov also works at the Centre for Discrete Mathematics and its Applications (DIMAP).
Spherical geometry makes the analysis of the grasshopper problem more complicated. Dmitry, being from the younger generation, used a 1948 textbook and pen-and-paper calculations, whereas I resorted to my good old Mathematica methods.
Mike Paterson, Professor, University of Warwick
The study titled “Globe-hopping” has been published in the Proceedings of the Royal Society A. This interdisciplinary study involves theoretical physics and mathematics, with applications to quantum information theory.
The researchers include Dmitry Chistikov and Mike Paterson (both from the University of Warwick), Olga Goulko (Boise State University, United States), and Adrian Kent (Cambridge). According to them, the next steps to offer a deeper understanding of the probabilities of quantum spin states is to search for the most grasshopper-friendly lawns on the sphere or even allow the grasshopper to bravely jump in three or more dimensions.
Journal Reference
Chistikov, D., et al. (2020) Globe-hopping. Proceedings of the Royal Society A. doi.org/10.1098/rspa.2020.0038.