May 13 2019
Cuprate high-temperature superconductors are examples of strongly correlated materials, in which superconductivity can be regulated either by altering the kinetic energy, or transfer energy, of electrons or by altering the number of electrons in the system.
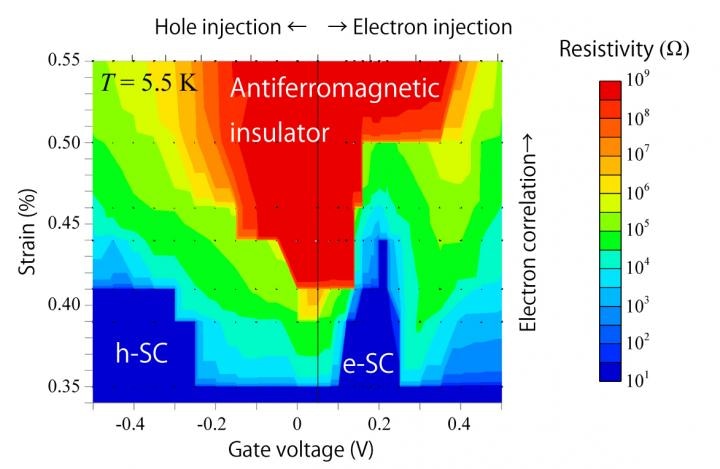 Resistivity is shown by colors. The insulator region (red) is surrounded by the superconducting regions (blue). The shapes of the insulating and superconducting regions differ between the negative and positive ranges of the gate voltage. The shape of the electron-doped superconducting region (e-SC) is found to be quite anomalous. (Image credit: NINS/IMS)
Resistivity is shown by colors. The insulator region (red) is surrounded by the superconducting regions (blue). The shapes of the insulating and superconducting regions differ between the negative and positive ranges of the gate voltage. The shape of the electron-doped superconducting region (e-SC) is found to be quite anomalous. (Image credit: NINS/IMS)
A huge number of strongly correlated materials have been analyzed with varied parameters to figure out the mechanism of superconductivity, but in spite of this, the range of parameter control is invariably restricted. As a result, an adaptable experimental technique to attain concurrent control of the transfer energy and the number of electrons has been preferred for a long time.
Now, a team of researchers at RIKEN, Nagoya University, Institute for Molecular Science (IMS), and Toho University has built a “correlated” transistor, or flexible electric-double-layer transistor (EDLT), made up of an organic strongly correlated material. While the EDLT substrate can be bent to control the transfer energy of electrons, the gate voltages of the EDLT can be used to control the number of electrons. The researchers observed that in both cases of decreasing and increasing the number of electrons, the system converted from an insulator to a superconductor. Yet, conditions for these superconducting states in these two cases were noticed to be profoundly different. Moreover, bending the substrate resulted in another superconducting state. The current result has been reported online on Science Advances on May 10th, 2019.
By utilizing a crystal of the organic strongly correlated material composed of BEDT-TTF (bis(ethylenedithio)tetrathiafulvalene) molecules, researchers successfully developed the EDLT. When the gate voltage was applied to the crystal surface, the electron numbers can either be decreased (hole doping) or increased (electron doping). Such an EDLT device is flexible, and when a mechanical force, or strain, from the rear side of the EDLT is applied, the transfer energy can be regulated. The investigators effectively regulated superconductivity in an analogous sample, and they did this by accurately altering the strain as well as the gate voltage.
The areas of superconducting states can be seen in the above image. The abscissa reveals the gate voltage that relates to the number of doped electrons, and the strain or mechanical force applied to the device through bending is shown by the ordinate. While the electrons are moving down along the ordinate, they are able to shift more easily due to the increased kinetic energy of electrons. The areas of superconducting states (blue) surround the area of the insulating state (red). A pair of superconducting regions of the right and left sides of the insulating region is considerably varied in shape, as shown in the image. In particular, the superconducting state that appeared with a growing number of electrons (the right side of the image) shows exceptional behavior, indicating that the state emerged abruptly when the number of electrons was slightly increased, and vanished when excess electrons were added. Increasing and decreasing the number of electrons can help obtain the superconducting states. Conversely, the features of the two states are observed to be significantly different.
Thus, the two-dimensional (2D) phase diagram was achieved with the help of a single sample. This diagram reveals the nature of the superconducting phase transition, which has been expected from the data obtained from numerous different samples prior to the appearance of this device. As a result, the recently created experimental technique speeds up to achieve the phase diagrams. More importantly, drawing the complete phase diagram from the same sample allows one to achieve more dependable results, irrespective of the impacts of difference and of impurity in crystal structures.
Such an experimental technique is applicable to numerous organic strongly correlated materials. One fascinating case is the quantum spin liquid, wherein the directions of electron spins are arbitrarily moving even at 0 K. Experiments conducted on this quantum spin liquid will expose the association between magnetism (arrangement of electron spins) and superconductivity. Moreover, it is indeed remarkable that the phase diagram of strongly correlated electron system is a considerable target of quantum simulators. The current result offers one potential standard solution for those recently developed calculation techniques.