Oct 10 2012
If quantum computers are ever going to perform all those expected feats of code-breaking and number crunching, then their component qubits---tiny ephemeral quantum cells held in a superposition of internal states---will have to be protected from intervention by the outside world. In other words, decoherence, the loss of the qubits' quantum integrity, has to be postponed.
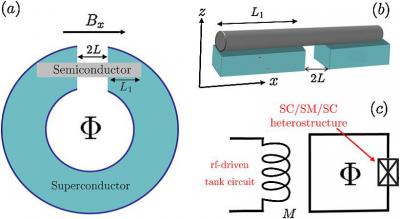 "Setup for Viewing Majorana Qubits
Picture of the prospective experimental setup in which Majorana particles could be made in a hybrid superconductor-nanowire material. In (a) the topological semiconductor bridges a gap between two parts of a superconductor. The letter phi represents an external magnetic field which can tailor conditions in the semiconductor. In (b) the immediate nanowire (gray rod) environment is shown. L is the size of the gap between the superconductor halves while L1 is the distance over which the underlying superconductivity will persist within the overlying semiconductor. (c) shows how the superconductor (SC)-semiconductor (SM) sandwich can be further tuned by a nearby electrical circuit. Credit: Lutchyn, Sau and Das Sarma"
"Setup for Viewing Majorana Qubits
Picture of the prospective experimental setup in which Majorana particles could be made in a hybrid superconductor-nanowire material. In (a) the topological semiconductor bridges a gap between two parts of a superconductor. The letter phi represents an external magnetic field which can tailor conditions in the semiconductor. In (b) the immediate nanowire (gray rod) environment is shown. L is the size of the gap between the superconductor halves while L1 is the distance over which the underlying superconductivity will persist within the overlying semiconductor. (c) shows how the superconductor (SC)-semiconductor (SM) sandwich can be further tuned by a nearby electrical circuit. Credit: Lutchyn, Sau and Das Sarma"
Now theoretical physicists at the Joint Quantum Institute (JQI) and the University of Maryland have done an important step forward to understand qubits in a real-world setup. In a new study they show, for the first time, that qubits can successfully exist in a so called topological superconductor material even in the presence of impurities in the material and strong interactions among participating electrons. To see how qubits can enter into their special coherence-protection program, courtesy of "Majorana particles," an exotic form of excitation, some groundwork has to be laid.
QUANTUM MATERIALS
Most designs for qubits involve materials where quantum effects are important. In one such material, superconductors (SC), electrons pair up and can then enter into a large ensemble, a supercurrent, which flows through the material without suffering energy loss. Another material is a sandwich of semiconductors which support the quantum Hall effect (QHE). Here, very low temperatures and a powerful external magnetic field force electrons in a thin boundary layer to execute tiny cyclone motions (not exactly, but ok—also isn't a cyclone a storm?). At the edge of these layers, the electrons, unable to trace out a complete circular path, will creep along the edge, where they constitute a net electrical current.
One of the most interesting and useful facts about these electrons at the edge is that they move in one direction. They cannot scatter backwards no matter how many impurities (which in ordinary conductors can lead to energy dissipation) may be in the material. If, furthermore, the electrons can be oriented according to their spin---their intrinsic angular momentum---then we get what is called the quantum spin Hall effect (QSH). In this case all electrons with spin up will circulate around the material (at the edge) in one direction, while electrons with spin down will circulate around in the opposite direction.
The QHE state is depicted in figure 1.
TOPOLOGICAL MATERIALS
In some materials the underlying magnetism of the nuclei in the atoms making of the material is so strong than no external magnet is needed to create the Hall effects. Mercury-cadmium-telluride compounds are examples of materials called topological insulators. Insulators (not sure how this sentence was supposed to start, but grammatically is currently confusing) because even as electrons move around the edge of the material with very little loss of energy, the interior of these 3-dimensional structures is an insulator; no current flows. The "topological" is a bit harder to explain. Partly the flow of current on the outside bespeaks of geometry: the electrons flow only at the edge and are unable (owing to quantum interactions) from scattering backwards if they meet an impediment.
But topology in this case has more to do with the way in which the motion of the electrons in these materials are described in terms of "dispersion relations." Just as waves of white light will be dispersed into a spectrum of colors when the waves strike the oblique side of a prism, so electron waves (electrons considered as quantum waves) will be "dispersed," in the sense that electrons with the same energy might have different momenta, depending on how the electrons move through the material in question.
The idea of electron dispersal is often depicted in the form of an energy-level diagram. In insulators (the left panel of Figure 2) electrons remain in a valence band; they don't have enough energy to visit the conduction band of energies; hence the electrons do not move; the material is an insulator against electricity. In a conductor (middle part) the conduction and valence bands overlap. In the QHE (right panel) electrons in the interior of the material also do not move along; the bulk of the material is an insulator. But for electrons at the edge there is a chance for movement into the conduction band.
Now for the topology: just as a coffee cup is equivalent to a donut topologically---either can be transformed into the other by stretching but not by any tearing---so here the valence band can be transformed into a conduction band (at least for edge states) no matter what impurities might be present in the underlying material. In other words, the "topological" nature of the material offers some protection for the flow of electrons against the otherwise-dissipating effects of impurities.
The marvelous properties of superconductors and topological materials can be combined. If a one-dimensional topological specimen---a nanowire made from indium and arsenic---is draped across a superconductor (niobium, say) then the superconductivity can extend into the wire (proximity effect). And in this conjunction of materials, still another hotly-pursued effect can come into play.
MAJORANA PARTICLES
One last concept is needed here---Majorana particles---named for the Italian physicist Ettore Majorana, who predicted in 1937 the existence of a class of particle that would serve as its own antiparticle. Probably this object would not exist usefully in the form of a single real particle but would, rather, appear in a material as a quasiparticle, an ensemble excitation of many electrons.
Some scientists believe that qubits made from Majorana pulses excited in topological materials (and benefitting from the same sort of topological protection that benefits, say, electrons in QHE materials) would be much more immune from decoherence than other qubits based on conventional particles. Specifically Sankar Das Sarma and his colleagues at the University of Maryland (JQI and the Condensed Matter Theory Center) predicted that Majorana particles would appear in topological quantum nanowires. In fact part of the Majorana excitation would appear at both ends of the wire. These predictions were borne out. It is precisely the separation of these two parts (each of which constitutes a sort of "half electron") that confers some of the anticipated coherence-protection: a qubit made of that Majorana excitation would not be disrupted by merely a local irregularity in the wire.
A recent experiment in Holland provides preliminary evidence for exactly this occurrence (***).
ROBUST QUBITS AMID DISORDER
One of the authors of the new study, Alejandro Lobos, said that the earlier Maryland prediction, useful as it was, was still somewhat idealistic in that it didn't fully grapple with the presence of impurities, a fact of life which all engineers of actual computers must confront. This is what the new paper, which appears in the journal Physical Review Letters, addresses.
The problem of impurities or defects (which flowing electrons encounter as a form of disorder) is especially important for components which are two or even one dimensional in nature. The same is true for the repulsive force among electrons. "In 3-dimensional materials," said Lobos, "electrons (and their screening clouds of surrounding holes) can avoid each other thanks to the availability of space. They can just go around each other. In 1-D materials, this is not possible, since electrons cannot pass each other. In 1D, if one electron wants to move, it has to move all the other electrons! This ensures that excitations in a 1D metal are necessarily collective, as opposed to the single-particle excitations existing in a 3D metal.
So, in summary, the new Maryland work shows that disorder and electron interactions, two things that normally work to disrupt superconductivity, can be overcome with careful engineering of the material.
"A number of important theoretical studies before ours have focused on the destabilizing effects of either disorder or interaction on topological superconductors," said Lobos. "These studies showed the extent to which a topological superconductor could survive under these effects separately. But to make contact with real materials, disorder and interactions have to be considered on equal footing and simultaneously, a particular requirement imposed by the one-dimensional geometry of the system. It was then an important question to determine if it was possible to stabilize a topological superconductor under their simultaneous presence. The good news is that the answer is yes: despite their detrimental effect, there is still a sizable range of parameters where topological superconductors hosting Majorana excitations can exist. That's the main result of our study, which will be useful to understand and characterize topological superconductors in more realistic situations."