Sep 4 2013
Physics undergraduate Jeysthur Ang has been awarded an Outstanding Undergraduate Researcher Prize (OURP) for research he carried out at CQT. He is the fourth student supervised at CQT to receive the National University of Singapore prize.
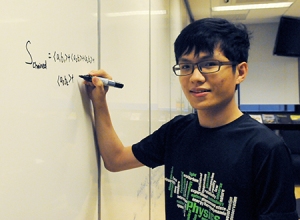Undergraduate Jesythur Ang won a university prize for his research with Valerio Scarani's group on certifying quantum randomness.
Jeysthur's research was on the certification of private randomness by Bell inequalities, a topic that has generated much recent excitement in the quantum information community. He was supervised by Valerio Scarani, a CQT Principal Investigator and NUS Professor. He also had a mentor who he met with weekly during the two-semester project: CQT PhD student Le Phuc Thinh, a former NUS undergraduate who was himself awarded the OURP in 2011.
"I would not have been able to make some (tiny) contributions to Physics without the supportive research culture of ConneQt, a research group within the Centre for Quantum Technologies. Hence, I would like to sincerely thank Professor Valerio Scarani for giving me the opportunity to work in his research group," says Jeysthur in his testimonial.
Prizes in common
"It is very heartening to see that our undergraduates continue to show exceptional potential in producing high quality research work. This is an affirmation of the Faculty's commitment in nurturing the potential of our undergraduates," wrote Andrew Wee, NUS Dean of Science, in a 21 June email announcing the OURP winners for the 2012-13 academic year.
Previous OURP winners who did their projects at CQT were Thiang Guo Chuan (2009-10) supervised by Berge Englert, and Le Phuc Thinh (2010-11) and Cai Yongqin Raymond (2008-09) supervised by Valerio. The OURP is awarded by the National University of Singapore to no more than 15 projects per year. Winners of the individual prize receive S$2000 and a certificate.
In 2012, Nelly Ng, then an undergraduate at Nanyang Technological University, won two graduation awards after completing her final-year research project under the supervision of Stephanie Wehner.
These other prize-winning undergraduate projects led to papers in peer-reviewed journals, and Jeysthur hopes his work will, too. With Thinh, Valerio and Research Fellow Lana Sheridan, he is writing up his results for publication.
Relaxing assumptions
The paper addresses an assumption commonly made in proposals to certify private randomness using the Bell inequality, a way to measure the quantum connection shared by pairs of particles. These proposals have attracted lots of attention because such guaranteed private randomness could find applications in secure communication, simulation and gaming.
When testing with a Bell inequality, a measurement must be performed on each particle of the pair. Typically the measurement is chosen from one of two options, and the original proposals for Bell-certified randomness assumed that this choice is made at random. This is called the assumption of 'measurement independence'. Loss of measurement independence affects the certification.
Jeysthur and his collaborators wanted to find out whether the certification would be more robust against the absence of measurement independence if the Bell inequality being tested were more general: in particular, if it involved more than two measurement options to choose from for each particle.
Unfortunately, the case they analysed didn't turn out to be any more robust than the standard setup. "The main result of my project is that we managed to consider the case for more than two inputs," explains Jeysthur.
Having now graduated with First Class Honours in Physics and a handful of other awards, including the Lee Kuan Yew Gold Medal for being the best performing graduate throughout the course of study for a Bachelor of Science degree, Jeysthur is now training at Singapore's National Institute of Education to become a teacher. His undergraduate studies were sponsored by the Ministry of Education.
CQT welcomes committed, talented undergraduates from all Singapore and international universities to apply for internships and research projects. Interested students should make enquiries directly to the Principal Investigator(s) in their area(s) of interest.