A feature of quantum physics known as entanglement arises when two or more systems interact in a way that makes it impossible to characterize each system's quantum state separately. They are described as entangled, or highly correlated, in the language of quantum physics. Quantum computing relies heavily on entanglement. The quantum computer becomes increasingly optimized and efficient with increasing entanglement.
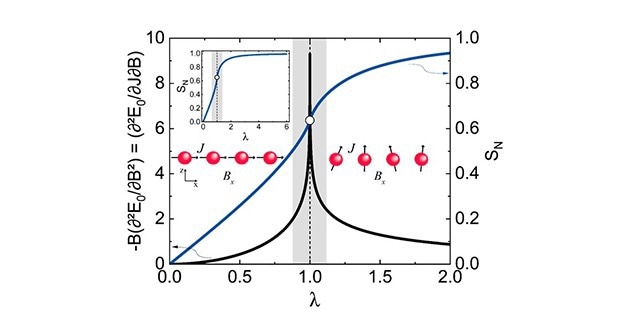This figure published in Physical Review B shows results indicating an increase in von Neumann entropy and a breakdown of the Hellmann-Feynman theorem. Explanatory details can be found in the original article. Image Credit: Physical Review B
In a study, the conditions for maximizing entanglement and a unique way of assessing it were examined. The researchers were connected to the Department of Physics at São Paulo State University’s Institute of Geosciences and Exact Sciences (IGCE-UNESP) in Rio Claro, Brazil. Optimizing the building of a quantum computer is one application.
The study’s publication appears as a Letter in Physical Review B.
The study demonstrated the situations under which the Hellmann-Feynman theorem falls. The theory, which is fundamental to quantum mechanics and is applied in fields ranging from particle physics to quantum chemistry, explains how a system’s energy depends on a control parameter.
Simply put, we propose a quantum analog of the Grüneisen parameter widely used in thermodynamics to explore finite temperature and quantum critical points. In our proposal, the quantum Grüneisen parameter quantifies entanglement, or von Neumann entropy, in relation to a control parameter, which may be a magnetic field or a certain level of pressure, for example. Using our proposal, we demonstrate that entanglement will be maximized in the vicinity of quantum critical points and that the Hellmann-Feynman theorem breaks down at a critical point.
Valdeci Pereira Mariano de Souza, Study Last Author and Professor, Institute of Geosciences and Exact Sciences, São Paulo State University
According to Souza, the findings advance fundamental physics research and could potentially impact quantum computing. Gordon Moore, a co-founder of Intel, noted that in 1965 he predicted that the number of transistors used in conventional computers would double every two years.
He stated that while quantum computing is advancing rapidly, thanks to recent technological advancements, with major companies like Google and IBM at the forefront, this rapid growth in the power of classical computers cannot continue.
de Souza added, “In conventional computing, binary language in terms of zeroes and ones is used to process information. Quantum mechanics, however, superimposes states and hugely increases processing capacity. Hence the growing interest in research on quantum entanglement.”
Souza came up with the idea and designed the study, and his postdoctoral researcher Lucas Squillante made significant contributions. The other members of the collaboration were Aniekan Magnus Ukpong (University of KwaZulu-Natal, South Africa), Luciano Ricco (University of Iceland), Roberto Lagos-Monaco (UNESP Rio Claro), and Antonio Seridonio (UNESP Ilha Solteira).
FAPESP funded the research that resulted in the publication of the study under projects 11/22050-4 and 18/09413-0.
Journal Reference:
Squillante, L., et. al. (2023) Grüneisen parameter as an entanglement compass and the breakdown of the Hellmann-Feynman theorem. Physical Review B. doi:10.1103/PhysRevB.108.L140403